Common Source Amplifier using FET - Engineering Projects

Common Source Amplifier

In this amplifier, input signal is applied between gate and source and the amplified output voltage is developed across a load resistor in the drain circuit. Thus, source is the common terminal between the input side and the output side.

Figure 1 gives the circuit of one stage of common source amplifier (CS) Amplifier using n-channel FET and with biasing arrangement. The typical component values are also shown in figure. This circuit is analogous to common emitter amplifier. On using p-channel FET, polarity of supply voltage is reversed. In the circuit of figure 1, R_s-C_s combination provides the self-bias.

Since no current passes through the reverse biased gate-source, the gate current I_G is zero. Hence d.c. gate voltage is,

$V_G = I_G \times R_g = 0$ ………..(1)

With d.c. drain current set to I_D, the dc voltage at the source is,

$V_S = I_D \times R_s$ ……….(2)

The gate source voltage is then,

$V_{GS} = V_G-V_S = 0-I_D\times R_s$ ……..(3)

Or, $V_{GS} = -I_D \times R_s$ …….(4)

However, quite often capacitor C_s is omitted from the circuit. Then resistor R_s no doubt provides the self-bias (caused by the flow of dc component of drain current) but also provides feedback from output circuit to the input circuit.

Analysis of Common Source Amplifier using FET

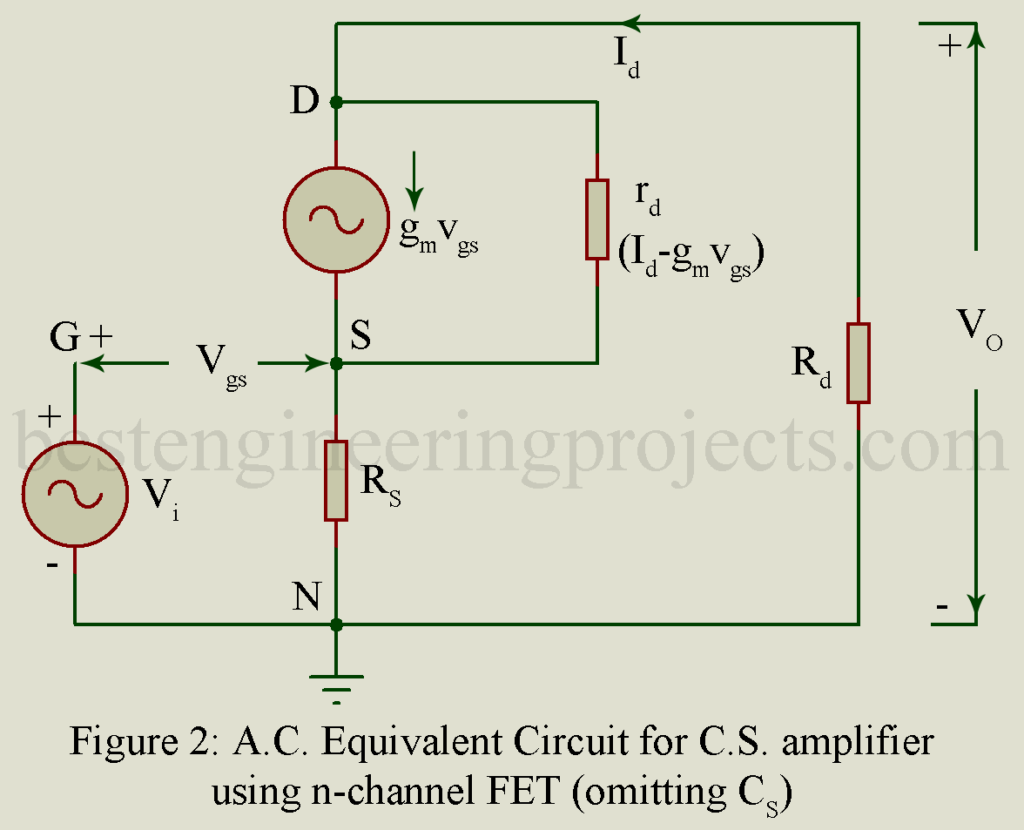
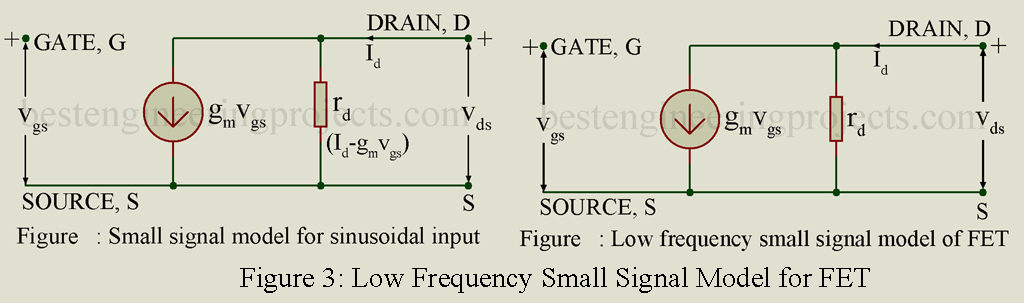
The analysis given below applies to both the n-channel and p-channel common source amplifiers. Figure 2 gives the ac equivalent circuit. Here FET has been replaced by its small signal model of figure 3.

On applying KVL to the output circuit we get

$I_d \times R_d + (I_d-g_m\times V_{gs}) \times r_d + I_d \times R_s$ …..(5)

From equivalent circuit of figure 2,

$V_{gs} = V_i-I_d\times R_s$ …..(6)

On substituting the value of V_gs from equation (6) in equation (5) we get,

$I_d = \dfrac{\mu V_i}{r_d + R_d + (\mu + 1)\times R_s}$ ……(7)

The output voltage V_o is developed across the load resistor R_d and is given by,

$V_o = -I_d \times R_d = \dfrac{-\mu \times V_i \times R_d}{r_d + R_d + (\mu + 1)\times R_s}$ ………(8)

The minus sign associated with V₀ as given by equation (8) shows that the output voltage V₀ is 180⁰ out of phase with V_i.

Hence voltage gain,

$A_v = \dfrac{V_o}{V_i} = -\dfrac{\mu \times R_d}{r_d + R_d + (\mu + 1)\times R_s}$ ……..(9)

Since the gate current is zero, input resistance is,

$R_i = \infty$ ………(10)

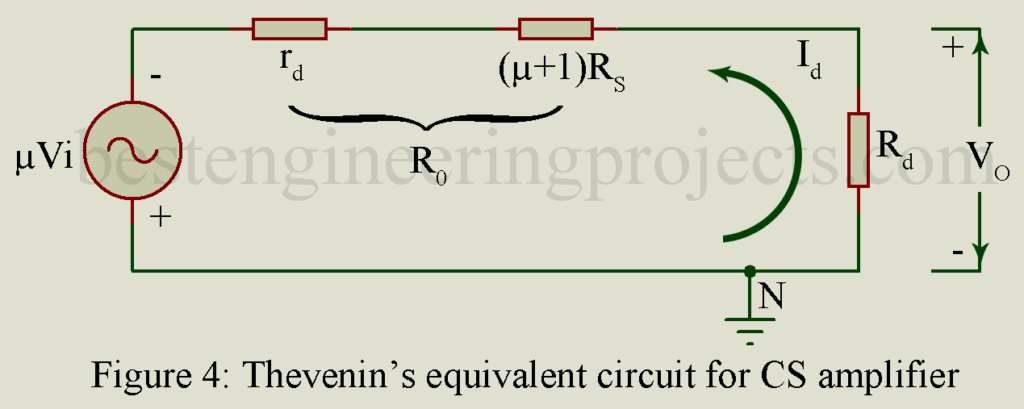
Making use of equation (8), figure 4 gives the equivalent circuit, called the Thevenin’s equivalent circuit, looking from load resistor R_d into the drain-to-ground N. Here (Thevenin’s) equivalent voltage is $-\mu \times V_i$ while its output impedance is,

$R_o = R_s \times (\mu + 1) + r_d$ ………(11)

With R_s = 0 In case, R_s is bypassed by a high value capacitor C_S i.e. if source S is effectively grounded, then in the above analysis, we may put R_S = 0.

Then from equation (9),

$A_V = \dfrac{-\mu \times R_d}{r_d + R_d}$ ……….(12)

$= -\dfrac{g_m \times r_d \times R_d}{r_d \times R_d}$

Or, $A_v = -g_m \times R_d^1$ ……..(13)

Where,

$R_d^1 = R_d || r_d = \dfrac{R_d \times r_d}{R_d + r_d}$

Output Impedance $R_o = r_d$ ………(14)

Numerical Problem on Common Source amplifier using FET

Example 1: A common source amplifier uses FET having dynamic resistance r_d = 120 k-ohm and $\mu$ = 20. Calculate the voltage gain and the output resistance R_o for the load resistance R_d equal to : (a) 300 k-ohm (b) 600 k-ohm and (c) 900 k-ohm.

Solution:

$R_o = r_d = 120 k\Omega$

Voltage gain, $A_v = \dfrac{-\mu \times R_d}{r_d + R_d}$

For R_d = 300 K-ohm, $A_v = -\dfrac{20 \times 300 \times 10^3}{(300 + 120)\times 1-^3} =-14.28$

For R_d = 600 K-ohm, $A_v = -\dfrac{20 \times 600 \times 10^3}{(600 + 120)\times 1-^3} =-16.66$

For R_d = 900 K-ohm, $A_v = -\dfrac{20 \times 900 \times 10^3}{(900 + 120)\times 1-^3} =-17.65$

Example 2: A common source amplifier uses loads resistance $R_d = 150 k\Omega$ and an un-bypassed resistor R_s in the source to ground circuit. the FET has drain resistor $r_d = 200 k\Omega$ and $\mu = 16$ . Calculate the voltage gain A_v and output resistance R_o for the following values of R_s: (i) $2 k\Omega$ (ii) $8 k\Omega$ (iii) $20 k\Omega$